Common Core and Problem Solving at the Alzar School
When the Common Core State Standards first came out, it was a somewhat controversial movement with its fair share of opposition. Slowly but surely, peer pressure, humility, and logic have gotten the better of 43 states and they have adopted the standards for Mathematics. At the Elementary School level, these standards stipulate what content is covered in which grade and promote deeper exploration of fewer topics. The pacing and structure of High School curriculum is still very much determined by the individual district. At this level, CCSS have accomplished two things:
The first is creating common language and titling regarding what content should be learned. Here’s what one of the content standards looks like:
CCSS.MATH.CONTENT.HSF.LE.A.4
For exponential models, express as a logarithm the solution to abct = d where a, c, and d are numbers and the base b is 2, 10, or e; evaluate the logarithm using technology.
It’s a very specific math skill. We teach this in both Pre-Calculus Functions and Algebra 2. It usually takes two days. High school math teachers in all but 7 states are using the exact same language, which can be instrumental in helping the math department at the Alzar School make a student’s transition to and from their sending school as smooth as possible and enabling them to not miss a beat.
But really, at the high school level, that’s only half of what the CCSS push schools to achieve with their students. There are over 100 different content standards for what students should learn in high school. The other half of the initiative with math standards are the mathematical practices standards. There are eight of them. These standards span all 13 grade levels and the topics taught therein. They are written in paragraph form and omit specific examples in order to maintain relevance to first-graders and Pre-Calculus students alike. But essentially, they collectively say one thing: Students must be able to solve word problems.
Students learning under the new common core standards are expected to express a practical situation using numbers and mathematical operations, then understand their solution in the context of the original circumstance. They are expected to be able to create a plan to solve a problem and defend the process they used. They will reflect on their own work, as well as the work of others. They will study patterns, analyze graphs, and develop their own problem-solving road maps, rather than just follow the ones we lay out for them.
Throughout this semester, students in all classes will work on POWs, or problems of the week. We have always strived to bring relevance in applications to the abstraction of algebra and now we are elevating these applications to a weekly exploration by students. Each problem will be carefully designed or selected to apply concepts we are studying in class and require some creative approaches from the students. We spent the first class day in every math class breaking down the following problem:
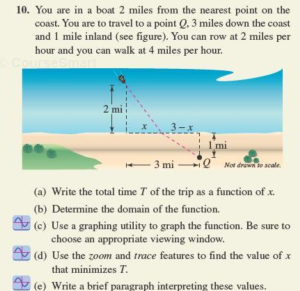
This problem involves relatively simple algebra: it incorporates the algebraic representation of a point on a number line, using the Pythagorean Theorem, and applying the relationship between rate, time, and distance. The problem-solving strategy is quite complex, which is why the problem provides a lot of guidance and why we solved the problem in class. For homework, students practiced using our POW format to show and justify their own work, and demonstrate their understanding of this problem. Here is an example of one student’s work:
Working out problems with this level of detail is not something I expect of students on a daily basis, just once a week. One of the powerful outcomes I already see is the level of feedback this provides me on student understanding. When students communicate their understanding so clearly, it makes it easy to identify strengths and misconceptions alike. These problems offer an incredible amount of partial credit. The scoring is based on understanding the problem, contextualizing and de-contextualizing, explaining algebraic work, developing a plan, making predictions, and reflecting. In this example, the student actually did not arrive at the correct answer. Her understanding, plan, work, and explanations are all clear and accurate. At the end, it’s likely that she misplaced a parentheses when entering a long formula into her graphing calculator. Precision in that final solution will be vital when she’s working in aerospace engineering, but we aren’t there yet. The precision is pointless if students cannot explain what x=2.25 means. This student earned 9.5 out of 10 for a wrong answer.
Stay tuned for some more problems of the week from the math department at the Alzar School. If you’re starting to feel some rusty wheels turning again from high school Algebra 2 class, take a shot at the POW some of our students will be contemplating over the weekend:


